Heat Transfer Problem

An engineer, you work for a consulting firm responsible for the renovation of old office buildings for a military base. A new regulation concerning wall materials goes into effect next year. The wall you are working on will not meet the new regulations. Conflict arises when there are differing opinions regarding which regulations to follow.
Questions on Ethics and Professionalism
Introduction
The design of a house wall as given in Figure 1. presents a common situation where it is useful to be able to calculate the heat transfer rate through a plane surface. Here, typical wood-frame construction is presented. Wood siding on the outside surface of the wall is followed by plywood sheathing. Glass mineral fiber insulation forms the main thermal resistance in the wall between the wood framing members (not shown). The inside surface is constructed of sheet rock.
Engineering Principles
The student should identify the number of thermal resistances in this wall. Are there four resistances? More? Less? What equation is used to calculate each resistance? Material properties and thicknesses are given below in Table 1.
(NOTE: The instructor may want the student to construct the thermal circuit at this point and make sure that the convection resistances on each side of the wall are included. Typical values are houtside = 34 W/m2K, hinside = 9.3 W/m2K. 1)
| Material | Thickness (cm) | Thermal Conductivity (W/m-K) |
|---|---|---|
| Wood | 0.95 | 0.14 |
| Plywood | 1.27 | 0.12 |
| Glass Fiber | 9.0 | 0.038 |
| Sheet Rock | 0.95 | 0.17 |
The student should know how to calculate the heat transfer rate through the wall for the given winter weather conditions: Tinside= 23 °C, Toutside = 15 °C.
One of the practical problems associated with house construction in moist climates is that moisture from the inside of the house (due to water vapor given off from cooking, washing, people) will tend to migrate from the inside to the outside. If the moisture condenses in the glass fiber it will degrade the effective conductivity of the material as well as cause other problems.
Calculate the point in the wall where the water will condense (if at all). If it does condense, will it cause a problem? Assume an indoor relative humidity of 50% and an outdoor relative humidity of 90%.
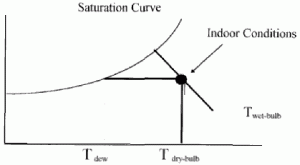
(NOTE: The student will need to review psychrometrics and determine the point in the wall where the temperature drops to the dew point temperature determined by the indoor drybulb temperature and the indoor relative humidity. The student will need to use the thermal circuit and determine the surface temperatures on either side of the glass fiber insulation. A psychrometric chart is shown below.)
Engineering Problem
You are an engineer working for a consulting firm responsible for the renovation of a series of old office buildings on a military base. Part of your assignment is to design the new wall construction for these buildings. You decide to add 9 cm (3.5 inch) of glass fiber insulation to the wood framed walls where no installation existed before. You know that one of the military specs with which you must comply states that all insulating materials shall be designed and installed such that no vapor condensation shall occur in the building walls as a result of the insulation design. This design process occurs in March and the new military specification will not become effective until January 1 of the following year. At your own initiative you decide to check if condensation will occur in the new wall construction of the buildings. (At this point the teacher may ask the students to do these calculations.)
Numerical Solution
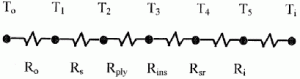
The equivalent electrical circuit for the thermal system is shown below:
The circuit equation for the heat flow is q = AT/Re
The equivalent resistance, Re, for the circuit is the sum of the individual resistances. The conduction resistances are given as Rcond = Ax/kA where Ax is the thickness of the material in the direction of the heat flow, k is the thermal conductivity of the material, and A is the surface area of the material perpendicular to the heat flow (a unit wall area of 1 m2 is assumed). The convection resistances on either side of the wall are calculated from Rconv = 1/hA, where h is the surface heat transfer coefficient and A is the surface area perpendicular to the heat flow. Thus, the six resistances can be calculated as shown below:
(Typical values are houtside = 34 W/m2K, hinside = 9.3 W/m2K.)
- Ro = 1/hoA = 1/[34(1)] K/W = 0.029 K/W
- Rs = Ax/kA = 0.0095/[2(0.14)1] = 0.0339 K/W
- Rply = Ax/kA = 0.0127/[~0.12)1]=0.106 K/W
- Rins = Ax/kA = 0.09/[(0.038)1]=2.37 K/W
- Rsr = Ax/kA = 0.0095/[(0.17)1]=0.056 K/W
- Ri = 1/hiA = 1/[9.3(1)] K/W = 0.107 K/W
- The sum of these resistances is Re = 2.70 K/W.
- The heat flow through the wall is q = AT/Re = [23 ( 15)]/2.70 = 14.1 W
- From circuit theory we know that the heat flow is the same through any resistance, or set of resistances, in a series circuit. To find the outer temperature of the insulation we can use,
- q = (T3 To)/(Ro + Rs + Rply) = (T3 ( 15))/(0.029+0.0339 + 0.106) = 14.1 W
- Solving we get T3= 12.6 °C.
We now need to check if the temperature of the insulation in the wall is below the dew point temperature of the air in the room. If so, the water vapor that diffuses through the wall will start to condense out in the glass fiber insulation. Returning to the psychrometric chart, using the indoor conditions of Tdrybulb = 23 °C and the Relative Humidity = 50%, we find that the dew point temperature is 12 °C. Since the lowest glass fiber temperature (12.6 °C) is below this dew point temperature (12 °C), water vapor will condense in the insulation and a problem does exist!
Ethical Problem
Your calculations show that moisture condensation will occur in the insulation in the walls during winter weather conditions. You share this with the engineering manager of your company and suggest that more expensive insulation (such as closed cell polyurethane foam) should be substituted for the glass fiber insulation. He states that the construction modifications should be completed by the end of November so the company will not need to comply with the new code. He also states that the cheaper insulation will save construction costs for his company. Therefore, he instructs you to proceed with current, less expensive design. What do you do?
Questions on Ethics and Professionalism
- What are the known facts and unknown relevant facts in this case?
- Discuss the legality of what the engineering manager is suggesting.
- Does it violate any professional codes (e.g. ASME, NSPE)?
- Does it violate the engineer's conscience?
- Develop positive and negative paradigms 2 as well as problematic cases that would fall between them.
- What are the possible choices of action for the engineer?
- What are the consequences of the possible solutions?
- Design alternate solutions to present to your engineering manager.
Ethical Solution
Known Facts:
- Vapor condensation will occur in the insulation for the current design.
- The condensation is in violation of future military specifications.
- Correction of the problem will require a more expensive insulation design.
- The engineering manager is probably not concerned with future military specifications, as they will not come in to effect before completion of project.
- Closed cell polyurethane foam will accommodate the military specs; however, it is a more expensive material.
Unknown Relevant Facts:
- Is the firm required by law to design in anticipation of future military specifications?
- Will the consulting firm contract with military base in the future?
- Is it likely that the project will extend into January of the next year?
- How much more expensive is the closed cell polyurethane foam?
- Will the additional cost of substituting polyurethane foam create an unprofitable job?
Legality
The manager is probably not in violation of the "letter" of the law, but he surely is in violation of the "spirit" of the military specification. A review of the complete military specification is in order.
Professional Code Violation
Relevant sections of the ASME/NSPE Codes are given below:
A. ASME 1.a. "Engineers shall recognize that the lives, safety and welfare of the public are dependent upon engineering judgments, decisions and practices incorporating into structures, machines, products, processes and devices." 1.b. "Engineers shall not approve or seal plans and/or specifications that are not of a safe design to the public health and welfare and in conformity with accepted engineering standards."
B. NSPE Code of Ethics of Engineers 1. The fundamental principles a. "..being honest and impartial, and serving with fidelity the public, their employers and clients." b. "...striving to increase the competence and prestige of the engineering profession." 2. The Fundamental Canons a. "Engineers shall act in professional matters for each employer of client as faithful agents or trustees, and shall avoid conflicts of interest" b. "Engineers shall build their professional reputation on the merit of their services....
Clearly the engineer should not "... approve or seal plans and/or specifications that are not of a safe design to the public health and welfare and in conformity with accepted engineering standards." One may argue that the "engineering standard" (in this case, the military specification) is not yet in effect, but this is a weak excuse.
- Does it violate the engineer's conscience? In general, an engineer should not be required to perform any act that violates his or her conscience. The engineer should not be expected to do something that directly contradicts his or her personal or religious beliefs.
Positive Paradigm
Design new insulation using closed cell polyurethane foam. The military assumes additional costs corresponding to new design. No vapor condensation occurs in walls.
Negative Paradigm
Renovation of office buildings using glass fiber insulation. Renovation is not complete prior to January. Condensation occurs as forecast. Possible litigation.
- Problematic Cases
- Design new insulation using closed cell polyurethane foam. Contractor assumes additional costs corresponding to new design. No vapor condensation occurs in walls.
- Renovation of office buildings using glass fiber insulation. Renovation is completed in November as projected. Vapor condensation occurs in walls.
Alternate Solutions:
The engineer might consider other alternatives such as using a special plastic vapor barrier against the interface between the sheet rock and the insulation. The engineer might have a frank discussion with the manager and explain that money is not the only factor here. The reputation of the company is at stake. If the government finds out what he is doing the company may never obtain any future contracts. This argument may be persuasive.
References
Authors: Wayne Helmer, Southern Illinois University, Carbondale, Illinois, and Robert C. Walker, Texas A & M University, College Station, Texas.
These problems were originally developed as part of an NSF-funded project to create numerical problems that raise ethical issues for use in engineering and other course assignments. The problems presented here have been edited slightly for clarity.